"You blow a methane bubble with a volume of 200.0mL at a pressure of 1.00 atm and a temperature of 20.0°C. Because methane is lighter than air, the bubble floats upward until it reaches and an altitude where the pressure is 0.600 atm and a temperature of -10.0°C."
- Using Boyle's Law we can calculate the new volume of the bubble just
due to the pressure change:
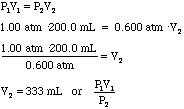
Sea Level High Altitude P1 = 1.00 atm P2 = 0.600 atm V1 = 200.0 ml V2 = ?
- Using Charles' Law we can take this new larger bubble due to the pressure
change and let it cool down due to the temperature change:
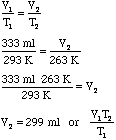
Warm High Altitude Cool High Altitude T1 = 20.0°C = 293 K T2 = -10.0°C = 263 K V1 = 333 ml V2 = ?
- Notice that the V2 from the first problem was substituted
for the V1 in the second problem. We can substitute this algebraically
as follows:

- Voila we have the Combined Gas Law! This can be used to solve any of the problems we have encountered so far, even those in which pressure, volume, or temperature do remain constant. In problems such as these the constant property will mathematically cancel out on each side.
-
Solving the above problem using the Combined Gas Law is as follows: 
Warm Sea Level Cool High Altitude P1 = 1.00 atm P2 = 0.600 atm V1 = 200.0 mL
V2 = ? T1 = 293 K
T2 = 263 K
previous page
chemsite homepage